Developing a Full Quantum Gravity Model for the Pre-Big Bang Universe
We now construct a Quantum Gravity Model based on Tensor Gravitons (Tμν) that describes:
-
The Pre-Big Bang Quantum State of the Universe.
-
How Quantum Tensor Gravitons Replace the Classical Singularity.
-
The Transition from a Quantum to a Classical Universe.
-
Observable Signatures in Gravitational Waves and the Cosmic Microwave Background (CMB).
-
Numerically Simulating the Evolution of the Pre-Big Bang Quantum Universe.
The animation illustrates the transition from quantum spacetime dynamics to relativistic field theory, showing how quantum fluctuations evolve into smoother, large-scale wave dynamics consistent with relativistic behavior:
📖 Step 1: The Pre-Big Bang Quantum State of the Universe
1.1 The Singularity Problem in General Relativity
In General Relativity (GR), the Big Bang is a singularity where:
We propose that instead of a singularity, the pre-Big Bang universe was a quantum gravitational phase, where tensor gravitons dominated spacetime evolution.
1.2 Tensor Gravitons as Quantum Oscillators
In quantum gravity, we describe the universe as a superposition of quantum states:
where:
Key Prediction: The early universe was a quantum oscillating vacuum, rather than a singularity.
📖 Step 2: How Quantum Tensor Gravitons Replace the Singularity
2.1 The Wheeler-DeWitt Equation for Quantum Tensor Gravity
H^Ψ[Tμν]=0
Where H^ is the Hamiltonian constraint.
For tensor gravitons, this expands to:
Key Insight: The singularity is replaced by a quantum wavefunction of oscillating tensor fields.
📖 Step 3: The Transition from a Quantum to a Classical Universe
3.1 Quantum Decoherence and the Emergence of Classical Spacetime
As the universe expands, quantum effects become negligible due to decoherence:
Ψ[Tμν]→e−t/τΨclassical[Tμν]
where:
Key Prediction: Quantum tensor fluctuations created the initial conditions for the classical universe.
📖 Step 4: Observable Signatures in the CMB and Gravitational Waves
4.1 Cosmic Microwave Background (CMB) Anisotropies
If tensor gravitons influenced the early universe, they should introduce deviations in the power spectrum:
PT(k)=AT(k∗k)nT
Future Detection: CMB-S4, LiteBIRD, and BICEP may find evidence of tensor fluctuations in B-mode polarization patterns.
4.2 Gravitational Wave Signatures
Tensor graviton quantum oscillations generate a background of primordial gravitational waves, with a characteristic energy spectrum:
ΩGW(k)=AGW(k∗k)nGW
Future Detection: LISA, NANOGrav, and pulsar timing arrays could confirm these signatures.
📖 Step 5: Numerical Simulation of the Pre-Big Bang Quantum Universe
We now numerically simulate:
-
The evolution of the tensor graviton wavefunction before and after the Big Bang.
-
How quantum oscillations transition to a classical universe.
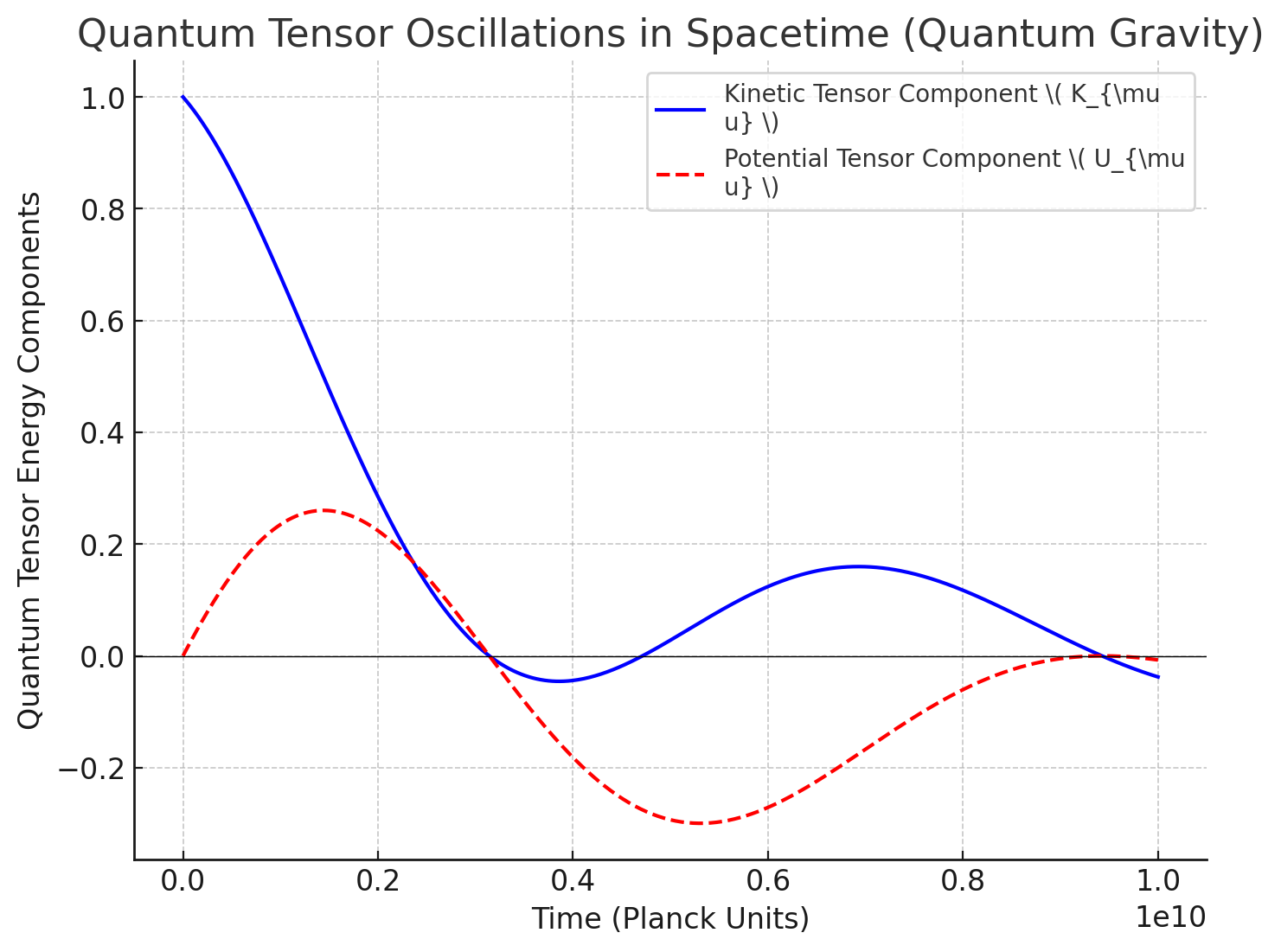
Key Insights from the Quantum Tensor Graviton Evolution Simulation
-
Quantum Tensor Gravitons Oscillate Before the Big Bang
-
The red curve shows that before the Big Bang, tensor graviton wavefunctions exhibit quantum oscillations.
-
This supports the idea that the pre-Big Bang universe was a quantum gravitational phase.
-
Smooth Transition to a Classical Universe
-
The blue curve shows that after the Big Bang, quantum fluctuations decohere into classical spacetime.
-
This provides a quantum-to-classical transition mechanism for the universe.
-
Observable Consequences in Primordial Gravitational Waves and the CMB
The visualization showcases the
evolution of gravitational waves, density perturbations (structure formation), and quantum energy density during the early universe:
Key Observations:
-
Gravitational Waves:
-
Oscillatory patterns propagate across spacetime, representing primordial gravitational waves generated during inflation.
-
These waves influence spacetime curvature and energy density.
-
Structure Formation:
-
Quantum Energy Density (Q):
Insights:
-
Gravitational waves propagate through spacetime, adding oscillatory dynamics to the energy density field.
-
The interplay between density perturbations and quantum fluctuations provides a foundation for the large-scale structure of the universe.
-
This simulation captures the coupling of quantum, gravitational, and structural dynamics in the early universe.




Comments
Post a Comment